13.4 Family Wise Error Rate (FWER)

Probability of making at least 1 type I error.

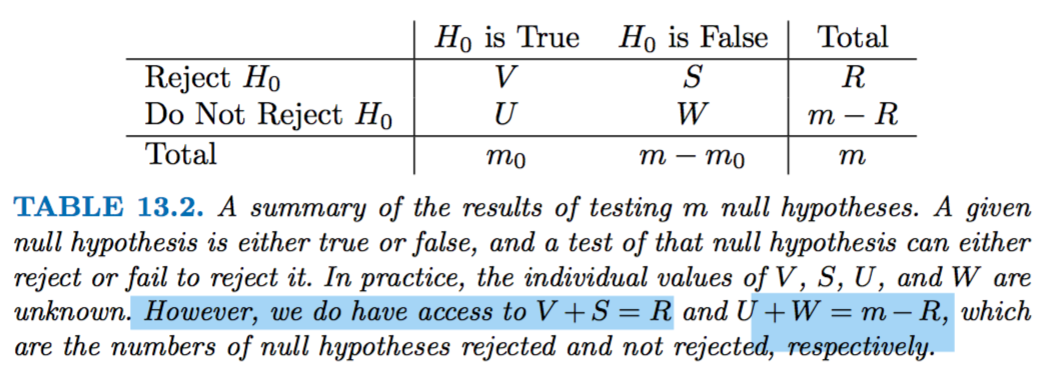
FWER is the probability of at least 1 type I error

$$FWER =Pr(V\geq1)=$$ $$=1-Pr(V=0)=$$ $$=1-\prod_{1}^{m}{(1-\alpha)}=$$ $$1-(1-\alpha)^m$$

With hypothesis that m tests are independents.

---

13.4.1 Controlling FWER

1. Bonferroni correction

$$FWER\leq m\frac{\alpha}{m}=\alpha$$ We set a new $\alpha$ value which is lower in proportion.

Bonferroni $\frac{\alpha}{m}=\text{new } \alpha$

2. Holm’s step down Holm’s $L=min{j:p_j>\frac{\alpha}{m+1-j}}$ is less conservative, with fewer type II errors and greater power.

$$\text{min }\{p_j>\frac{\alpha}{m+1-j}\}$$

These other two are subsequent methods to apply for further investigations:

3. Tukey’s method

4. Scheffè’s method

---